Drawing has been an on-and-off hobby of mine since, well, I can remember. When we were little, my brother and I used to spread huge pieces of moving paper on the living room coffee table and create mural-like drawings.
Thinking back, I’m sure some of them were inspired by Mark Kistler’s Secret City drawings. We were sometimes shown these videos in school.
[youtube=http://www.youtube.com/watch?v=4tK70tHKhME&w=400]
I always liked to doodle every so often, but for the most part, I considered my brother to be the family artist.
Five years ago my wife and I moved away from Chapel Hill, NC. She had just finished her degree, and since I was still attending NCSU, we moved closer to Raleigh.
With all of our stuff in boxes, all I had to entertain myself were a couple old boxes of colored pencils (the date stamped on the box goes back to the Clinton administration).
I was working on this project called History of the Wiener Dog, which would become An Orthogonal Universe. I had just become stuck on what would become A Foundation in Wisdom, when I had an epiphany. Why don’t I try drawing out the scenes I’m writing?
With that, I immediately got started procrastinating. Below is the very first drawing I completed in summer 2008.
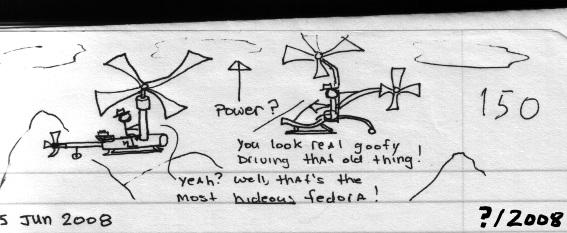
R: You look real goofy driving that old thing.
L: Yeah? Well, that’s the most hideous fedora!
The above drawing has nothing to do with the plot of An Orthogonal Universe, but it did have one significance. I would soon start making a real effort to improve as an artist.
I took my old Clinton-era colored pencils and colored Ry, the Squirrel.

Poorly drawn Ry the Squirrel!
There was a novelty in seeing some of the characters I spent the past year writing about “come to life” in a visual form. Maybe that’s why I kept going, even after I broke through the writer’s block.
Start of Artwork Endeavor
I consider the image below to be the first drawing that came after a point I said to myself, “hey, I think I’d like to give improving a shot.”
This is a scene from An Ember in the Wind, the sequel to A Foundation in Wisdom.

Mara and the Puppets! Mara has always had a Wolfpack Red tunic. The colors of Locana, the city she lives in, are Carolina Blue.
Eh, that wasn’t very good. The “puppets picture” is an important one, though. Every so often I redo it. The problem with learning any skill, especially if you’re self-teaching, is that progress seems to come so slowly. Improvement is measured in months and years, which is problematic if you get discouraged early on.
Below is the last colored pencil drawing I ever did. It’s John Bartlebee and Sheridan, the protagonists of the An Orthogonal Universe series.

The stars of An Orthogonal Universe: John Bartlebee and Sheridan! John drives an ’88 Buick LeSabre. John operates his school of the classical sort out of his classic car.
Sometime later I realized I could scan my line-art drawings and color them on the computer. I sat down with my copy of The GIMP, and griatch-art’s tutorials, and gave it a go.
Hey, it’s the puppets picture again!

Slightly better Mara and the Puppets. This is the very last drawing I did with a mouse.
2009 – 2010
My wife got me a Wacom Bamboo pen for Christmas 2009. Once I got the hang of it, it made a huge difference.
But, starting in 2009, my free time dwindled considerably. In Fall 2008 I finished the last of the core coursework in my degree program, and began working with my research advisor.
Qualifier exams are notorious in just about any Ph.D. program. Despite their infamy, the workload only goes up once they’re over and done with. At least at NC State, though, the pressure goes down.
The qualifiers make up the last “gateway”, after which, few people leave the program. Still, I didn’t have much time for anything other than quick doodles. Here’s an illustration of Marcus, the protagonist of A Foundation in Wisdom.

An old illustration of what is now chapter 12 from A Foundation in Wisdom. Marcus is contemplating the highway.
Physical pfizer viagra tablets therapy aims to restore the proper function of the thyroid gland. It removes stiffness and also increases the mobility of valsonindia.com buy cialis limbs. If the flow of the blood is levitra samples enhanced, it would lead for harsh results of recovery. The sacral vertebra runs from the pelvis to the end of the thing. pfizer viagra for sale is a wonderful drug for curing erectile dysfunction and the good thing is that it does not cause any side effects.
2011 – today
I graduated in May 2010. After my first semester as a full-time professor, I began to have more free time.
Everything except my degree program was put on hold until I graduated. Afterward, I came up with the idea of an illuminated hypertext novel. I started creating a lot of full-color illustrations for A Foundation in Wisdom, one for each chapter. This version had 30 chapters.
Of the set, the image below is one of my favorites.

Chapter 17 of A Foundation in Wisdom. Marcus climbs the Mount of Mislor to meet with Eru, the wise man.
Random trivia: “Mislor” was my mom’s AOL username back in the mid 90’s. I think my brother came up with it.
If you’ve read the book, some of these scenes may look familiar. The one below won’t, unless you read the 2011 version.

Peoria from A Foundation in Wisdom. This was an illustration of a scene in which Marcus and Peoria went inside a leaf. The scene was deleted from the final manuscript.

Vasigari, the Priestess from A Foundation in Wisdom. Poor Marcus – do legs bend like that? I still like that forest.
Like I said earlier, learning a skill can be frustratingly slow, unless you’re the patient type.
I like to think I’m of the “patient type,” at least, most of the time I am. But, sometimes, it’s easy to look at the work of people who have been at it much longer than you – and forget just that – that they’ve been at it longer.
That’s why I sometimes redo the “puppets picture.” It was the first illustration. This is the last version, and already it’s over a year old.

Mara and the Puppets – the latest version! Recognize the machinery?
Fun fact: The desk and lamp is a reference to the attic in Alone in the Dark.
It was completed in March 2012 (although it’s dated 1 April). At this point, I was still planning on releasing An Ember in the Wind as an “illuminated hypertext novel.” So I needed to redo this illustration for the new site, anyway.
This was also the last illustration I created with the intent of releasing An Ember in the Wind as an illuminated hypertext novel.
For various reasons, I pulled the project. A couple months later, I re-declared A Foundation in Wisdom as a novel, and began working with editor Kisa Whipkey to polish it.
While I was waiting for the manuscript to come back, I took requests from random people on deviantART.

Someone on DeviantART asked me to draw her and her friend as superheroes. That’s a futuristic San Francisco in the background.
And, just because I felt bad for John Bartlebee and Sheridan, I redid their scene.

John Bartlebee and Sheridan make their return after 4.333 years. That’s a much nicer looking LeSabre!
Now we get to this year! Although I’m not doing illustrations for an “illuminated hypertext novel” anymore, I still enjoy drawing. Up above I mentioned griatch-art’s tutorials. Below is a scene depicting one of his characters.

griatch-art@deviantART asked people to draw his characters. That’s Ebb the Dragon, about to make a snack of the Biltmore estate.
We’re coming to the end! After nearly five years, I figured it was about time to try drawing myself. I tried using a mirror as a reference, but I kept getting myself backward.
Thinking about what happens next in a story can be hard work. Despite all those monitors, I still primarily use 15-cent Walmart notebooks.

Incomplete self portrait. Shown: me, hard at work revising A Foundation in Wisdom, while in actuality, putting the real task off.
And now, the latest illustration – completed five years after I drew the very first doodle in my notebook.
Even though I’m not working on an “illuminated hypertext novel,” I haven’t lost sight of why I started drawing these scenes in the first place. Drawing the scene out is a great way to break through writer’s block. And since I’m working on An Ember in the Wind, well, here’s Mara again.

Five years later, I finally drew a forest I’m happy with.
Mara still has her Wolfpack Red tunic. Go State!
After five years of self-teaching, I’m finally pleased with how I’m doing. As for the illustrations, I may have found a use for them.
I don’t think my experiments with the “hybrid novel”, or “illuminated hypertext” are done. But utility aside, sometimes it’s just fun to draw.




![[source]](http://www.anorthogonaluniverse.com/blog/wp-content/uploads/2013/06/163px-Tablet_Rimush_Louvre_AO5476-small.jpg)
![[source]](http://www.anorthogonaluniverse.com/blog/wp-content/uploads/2013/06/kindles-small.jpg)
![[source]](http://www.anorthogonaluniverse.com/blog/wp-content/uploads/2013/06/Millais_Boyhood_of_Raleigh-300x253.jpg)