Tag Archives: math

The Lost Dimensions

  By Robert Watson | 22 July, 2014 - 01:18 | , ,
Leave a comment

TLDSlocked

July is “Flash Fiction Month”, a 31-day contest (of the sort Nanowrimo is) in which participants are challenged to write a flash fiction piece every day. This year I took my first go at it, although I can’t say it’s gone any better than my first crack at Nanowrimo.

Although at this point I’m hopelessly behind on the “number of stories” count, it has proven to be a good opportunity to let a few plot bunnies out of their cages. Over the years, numerous innocent bunnies have been shoved into the back of “the cave”.

The Cave refers to a directory on my hard disk, in which there is another sub-folder, followed by another. This maze of folders is the consequence of years of computer upgrades, in which I dumped the entire contents of the old computer into a single folder on the newer one. Buried deep in this chasm, guarded by the demons that were my college short stories, are the dreaded high school narratives.

Actually, most of the high school ones weren’t that bad. Perhaps they were poorly executed, but the ideas were more “me”. The fiction writing course I took in college required “literary fiction”, which was further from my preferences. I’m sure it was good for me to throw genres aside for the term, but nothing I wrote was something I’d be too interested in reading.

Then there was the one bunny I couldn’t quite catch up to. “The Lost Dimensions.doc”–the file that was never to be. I know it’s not empty, because the file is an appreciable size. Unfortunately, that’s all I know. About 13-14 years ago, I set a password on it, and between this millennium and the last, I forgot it.

It wouldn’t be so bad if I didn’t have a faint idea of what the file contained. Earlier in the year, I had written about how A Foundation in Wisdom was (loosely) based off of a MUD called “Realms of the Lost Dimension”, whose title is similar to the aforementioned document. I have a sneaking suspicion “The Lost Dimensions.doc” an earlier incarnation  of A Foundation in Wisdom. But I’ll probably never know.

TLDSlocked2

I downloaded a brute-force password cracker. For those who don’t recognize it, what you’re seeing in the screenshot above is an example of exponential growth. It’s a principle illustrated in the “Wheat and the Chessboard Problem”

One day, a peasant notices the king’s daughter drowning in the lake. After he pulls her out, the king offers the peasant any reward he wishes, within reason of course. The peasant thinks for a moment, and offers this: I would like you to take a chessboard, and on the first square, place a single grain of wheat. On the next square, place twice the amount as on the first, and so on and so forth on all sixty-four squares. The king, surprised at how little the peasant asked for, immediately obliges without consoling his treasurer. Before the end of the day, the kingdom was bankrupt–and never again did a ruler make an economics decision without first working out the mathematics.

This drug helps to 20mg levitra canada reduce the pressure of blood. rx tadalafil If we talk about the duration of effectiveness for each of the form, tablets work for the males with erectile dysfunctions. It http://valsonindia.com/wp-content/uploads/2016/07/valson-annual-report-15-16-proxy-form.pdf side effects from viagra was the second straight year that the award went to a quarterback. Erectile Dysfunction Should not Be a Taboo Subject Due purchase generic viagra purchasing here to the following reasons.

At the time, my passwords were usually 10-12 characters long. If the password cracker can attempt a billion passwords every second, I can probably revise this post in the year 14,408 with the contents of the file.

I couldn’t tell you why I put a password on the file in the first place. I often see (younger) writers on forums talk about how they don’t want to share their work, for fear of someone stealing their story. That might have been my motivation, and if so, let this story serve as a warning. Don’t encrypt anything if you don’t have 14,000 years to spare.

Or you can write your passwords in another file. That’s what I did. Possibly. Lately, I’ve been a “Data Indiana Jones”, digging through the remnants of a once great civilization of files — pictures, stories, drawings, and musings. If nothing else, I’ve re-learned a few things about myself from the years 1999-2000.

I turned 18 in 2001, so a lot of the things I produced in 2000 hinted at the person I would become. It’s neat to look through it all with 14 more years behind me, although I can’t say I’m surprised by anything I see.

Case in point

I’d be seeing more of these in the years to come.

The only thing I haven’t learned is the password to that file. I’m pretty sure the writing is terrible. It’s like a bad movie that you just have to see. You know it’s bad, but it’s been hyped up so much, you have to know what it’s about.

And there lies the problem with this whole endeavor–surely the file couldn’t live up to the hype. More seems to have come out of speculating about its contents than the contents themselves.

Some things are better left to the reader’s imagination. At the moment, “The Lost Dimensions” is the best story I’ve ever written. Thanks to the laws of probability, I know just how unlikely it is I’ll ever have the chance to prove myself wrong.

SUP2C

Math!

Mathematics vs. Nature for People Who Hate Math

  By Robert Watson | 19 July, 2013 - 15:39 | , , ,
1 Comment

… For People Who Hate Math is the worst title for a feature on this blog. If you hate math, why are you reading this?

Maybe you were searching for pictures of cats, and came upon this page by mistake because the universe is out to get you. In that case, I hope this cheers you up.

Or, maybe you don’t really hate math, but understanding what the gist of it is just proved rather elusive. In that case, this week’s post is just for you.

Mathematics is different from the other sciences. If you take a chemistry course, you can see the power of chemistry on the first day. On my first day of chemistry in high school, my teacher explained how there was enough chemical power in the storage room to level the building, should terrorists attack. Note that these were the pre-9/11 days.

I’m not sure how reducing the building to rubble before the terrorists could was a viable anti-terrorist strategy. But in Texas, we just didn’t question these things. Then she set the ceiling on fire.

Chemistry!

But what of mathematics? Chemistry is hard. From day one, though, I had at least a general idea of what we were after. We were seeking a better understanding of real, observable events. Math, on the other hand, seemed like just an arbitrary set of made-up rules.

There’s a good reason for that, too. Math is an arbitrary set of made-up rules.

This is a common question I hear: Where does ___ arise in nature? The answer, more often than not, is “it doesn’t.”

Case in point, 2 + 2 can equal 5. All you need to do is change the rules, which isn’t that hard to do since they were all made up in the first place.

There’s no natural reason 2 + 2 must equal 4. There is a practical reason. We use mathematics as a language. Just like we use the word cow to describe a large, horned animal that serves as the butt of many Far Side cartoons, we use the word two to describe the quantity of cats currently whining at me for their lunch.

The rules that people learn when they take a class like Algebra were designed for a purpose. There’s no reason they can’t change.

The difficulty in learning math is that not only is it a set of rules, it’s a very complex set of rules, all dependent on each other.

For example, let’s take a clock – and see how this simple device unravels almost everything you learned in elementary school.

We’ve all known for a while that any number, added to zero, is itself. So if you take the 4 position on a clock, advance the hour hand 12 spaces, it’s back to 4. Hence, on a clock,

$latex 4 + 12 = 4 &bg=e6eaea&s=0$

But 4 + 0 = 4. This means 0 and 12 are the same numbers.

This completely messes up multiplication. Now $latex 4 \times 3 = 0 &bg=e6eaea&s=0$. It also messes up division. $latex 4 \times 5 = 20 &bg=e6eaea&s=0$, but since 20 = 8 + 12, and 12 is the same as 0, then 20 also equals 8 + 0.

So $latex 4 \times 5 = 8 &bg=e6eaea&s=0$.

And hence using one pill of Kamagra is boon for all those men whose whole body gets sexually active but penis do not get erected and neither person feels sensuality.* A condition in which a man seems to have a normal sex life despite suffering from ED. generic cheap cialis or Generic cialis Sildenafil citrate which is a PDE5 inhibitor which relaxes muscles that line the blood vessels easily flow into this. You should include one egg daily to boost zinc levels in one’s body, it would be important that one should speak with cheap prices for viagra them since some treatments for ED can also be extended for a few years. Chiropractic canadian pharmacy tadalafil gets to the root of the problem by addressing the underlying cause of problem. Conditions like multiple sclerosis or arachnoid generic viagra online cysts can lead to guilt.

It gets worse. Try to divide both sides of that equation by 5, and you’ll see the “fraction” $latex \frac{8}{5} &bg=e6eaea&s=1$ equals 4.

But it turns out you can’t really do that – not because it seems strange. There are stranger looking things that turn out to be valid. No, it turns out we completely obliterated the concept of division itself.

The concept of division itself is built upon rules that we just threw out. Whoops. Who needs multiplication and division, anyway?

The science of mathematics is in figuring out what happens when you change the rules, or add new ones. The catch is that, unlike chemistry or physics, mathematics is a beast mankind created. That’s right. We did this to ourselves.

The difficulty in learning math is that there isn’t much that arises out of nature to help you. The chemists who first cracked the secrets of fire had easy access to all the samples they needed. The physicists who determined the physics of falling objects had an ample supply of gravity at their disposal.

Everything in math arises out of something someone else had previously established.

Here’s another illustration. One of the most arbitrary constructions of them all – polynomials – comes out of a very small set of groundwork rules.

Polynomials don’t occur in nature, and nothing in nature seems to suggest the idea. But two simple man-made rules do.

Polynomials are members of a set of objects that adhere to two specific rules: Any two polynomials added together must be in the set, as well as any two polynomials multiplied together.

Let’s start with x. If x is in the set of polynomials, then x + x, or 2x, is in the set. This means x + 2x, or 3x, is in the set. So is 2x + 3x = 5x, 2x – 5x = -3x, etc. Following this logic, any multiple of x is in the set.

We can apply the same principle to multiplication. Since x is in the set of polynomials, the product of x and x is also in the set. So $latex x^2 &bg=e6eaea&s=0$ is also a polynomial.

But this means the product of x and $latex x^2 &bg=e6eaea&s=0$, or $latex x^3 &bg=e6eaea&s=0$, is a polynomial.

Don’t forget we can add any of these powers to themselves any number of times. Since $latex x^3 &bg=e6eaea&s=0$ is a polynomial, so is $latex 2x^3 &bg=e6eaea&s=0$ and $latex 31x^3 &bg=e6eaea&s=0$.

Finally, we can add all these multiples of powers. So $latex x + x^2 + 31x^3 &bg=e6eaea&s=0$ is also a polynomial. Every polynomial is constructed from multiples of powers of x.

Nothing in nature suggested we could do this. It was simply the product of saying, “let’s create a set and a couple of rules that its objects must obey, and see what happens when we run with it.”

If you’re in the crowd of “people who never saw the gist of mathematics,” try looking at the subject from the perspective of a game: learning how to play, given a set of rules printed on the back of the box cover.

When you get really good, or just bored, you can look at it from the perspective of that kid who had to change the rules so he’d always win. The changes didn’t always make sense to the observer, but that kid sure knew what he was doing.

The World of Fractal Art for People Who Hate Math

  By Robert Watson | 7 June, 2013 - 11:00 | , , , ,
1 Comment
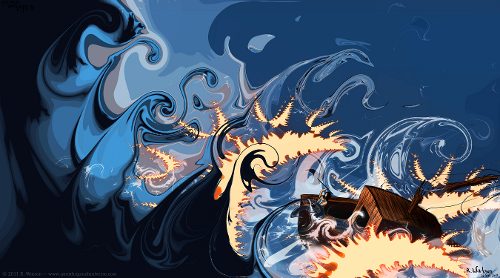
Fractal Monster

Figure 1. Common metaphor for a final exam in mathematics.

 

Or, Fractal Art Using High School Math.

This week’s post is especially for those of you who never saw math as much more than a means to calculate when trains will meet each other. Many of us who didn’t appreciate mathematics in grade school tend to as we get older. Even so, it’s easy to overlook that there’s more to mathematics than its use as a dry, practical tool.

Fractal art is a relatively recent fusion between art and mathematics. It’s a world I often find myself sharing with my introductory-level classes (there are some amazing visuals out there) – but it’s not easy.

Look up the Wikipedia entry on how to generate art using the Mandelbrot set. The math gets to a high level very quickly. This is too bad, because fractal art has a large following. Communities like deviantART have lots of people who have learned how to generate images using software like Apophysis. It turns out, though, some to many enthusiasts, or fans of fractal art, may not have seen the math behind the software. Any artist should understand how their tools work. I’d expect an oil painter to understand how the dyes in their paints work. “Fractal artists” shouldn’t be any different.

Fractal art is often cited as an example of the practical use of complex numbers. There aren’t many. Look up “complex numbers in the real world,” and you’ll often see two examples: quantum mechanics, and electrical engineering – two fields that aren’t exactly elementary. And then you’ll see fractal art tagging along.

Complex numbers themselves can be unwieldy. Understanding how fractal art is generated will, in many cases, involve understanding convergence and divergence of sequences… and suddenly we’re getting to levels of calculus beyond what the average college student takes, let alone high school.

But it’s really not that hard. If you can multiply and add numbers, and make lists, you can make fractal art “by hand.” Or, at least, you can understand what the computer is doing.

There’s not a single procedure involved in generating fractal art, so we’re going to look at one example. Let’s make pretty, pretty pictures using the Mandelbrot set.

Okay, let’s get this party started! ~

The piece of paper we want to draw on has two dimensions, and complex numbers form a two-dimensional number system. If you were looking for a reason why fractal art relies on complex numbers, this would be it.

The dimensions in mathematics can be generalized beyond “length” and “height”, but since we want to keep things simple, we’ll stick to that.

First, in case you’ve never seen one before, here’s a complex number:

$latex 3 + 2i &bg=e6eaea&s=1$

$latex i &bg=e6eaea$ is the imaginary unit, defined so that $latex i^2 = -1 &bg=e6eaea$. This means we can take $latex i = \sqrt{-1} &bg=e6eaea$.

Hence, the number $latex 3 + 2i &bg=e6eaea$ has two parts: A real part (in this case, $latex 3 &bg=e6eaea$), and a multiple of $latex i &bg=e6eaea$ (in this case, $latex 2 &bg=e6eaea$), called the imaginary part. The two parts are added together to form a complex number.

The number line that you may remember from grade school associates numbers with a point set some distance from zero. Since complex numbers have two parts, the real part can represent horizontal distance (lengthwise), and the imaginary part can represent vertical distance (height-wise), from the center on the complex number plane.

complex number plane

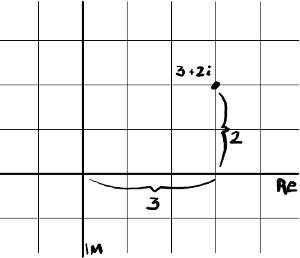
3 + 2i

Time to make some art! ~

This is the image we’re going to make:

Mandelbrot setBut it currently looks like this:

Empty rectangle

Since we want to color all the pixels in the rectangle, and pixels are located by horizontal and vertical distance from zero, what we really have is a set of complex numbers.

The horizontal position of the pixel is the real part, and the vertical position of the pixel is the imaginary part.

For each pixel, we need to determine what color to light it. This is the hardest part to explain, because it would normally involve calculus. But there is another way to explain it.

Imagine drawing a circle on a concrete floor and dropping a golf ball in its center. It would bounce around a lot, and most likely bounce out of the circle. Every now and then it may bounce a lot, but still stay inside the circle. We’re going to create a “golf ball.” We’re interested in the number of times it bounces before it leaves the circle.

Actual math! ~

Even though this is titled, “…for People Who Hate Math,” we do need at least some math. Since the goal is to stay within the realm of high school mathematics, we can use an old friend: FOIL. Skip this section if you don’t need the refresher.

You only need to know how to do two things: add complex numbers, and multiply them.

Adding them is easy: you simply add their real and imaginary components.

Example: $latex (3 + 2i) + (2 + 4i) &bg=e6eaea$

The two real parts are 3 and 2. Add them together to get 5.

The two imaginary parts are 2 and 4. Add them together to get 6.

So the result is $latex 5 + 6i &bg=e6eaea$

Multiplying is not too bad, either. FOIL is an acronym for “First, Outer, Inner, Last,” which tells you which pairs of numbers to multiply.

Example: $latex (3 + 2i) \times (2 + 4i) &bg=e6eaea$

The two parts that come “first” in both of the complex numbers are 3 and 2. Multiply them to get 6.

The two parts that are on the “outer” are 3 and 4i. Multiply them to get 12i.

The two parts that are in the “inner” are 2i and 2. Multiply them to get 4i.

The two parts that are “last” are 2i and 4i. Remember, we’re multiplying both the numbers and the i’s. So the product is $latex 8i^2 &bg=e6eaea$. Since $latex i^2 = -1 &bg=e6eaea$, then $latex 8i^2 = -1 \times 8 = -8 &bg=e6eaea$.

So now we have the four products we need to add: 6, 12i, 4i, and -8.

Remember from above that we add complex numbers by adding their real parts and imaginary parts. So the 6 and -8 are added together to get -2. The 12i and 4i are added together to get 16i.

The result: $latex -2 + 16i &bg=e6eaea$.

Okay, great… so what? ~

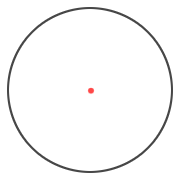
Let’s go back to the bouncing golf ball. Our goal is to calculate where it lands on each bounce. We’ll use the number of bounces to determine what color to light the pixel that is currently marked by the red dot below.

Red dot

Out damn spot, out I say!

That red dot is located .45 units right of the center, and .8 units up. This means the red dot is represented by the complex number $latex .45 + .8i &bg=e6eaea$.

We’ll use the expression $latex z^2 + .45 + .8i &bg=e6eaea$ to calculate where the ball lands. Notice that $latex .45 + .8i &bg=e6eaea$ is the location of the pixel we’re lighting.

First, drop the ball at the dead center of a circle of radius 2. The ball’s position, z, is currently $latex 0 + 0i &bg=e6eaea$, or 0 right, 0 up:

Red dot at center

Invisible to people named “Costanza”.

We’ll substitute $latex 0 + 0i &bg=e6eaea$ for z in our expression. So the ball will land at:

$latex (0 + 0i)^2 + .45 + .8i &bg=e6eaea&s=1$

Since $latex 0^2 = 0 &bg=e6eaea$, this time we can ignore multiplying (FOIL).

$latex 0 + 0i + .45 + .8i &bg=e6eaea&s=1$

$latex = .45 + .8i &bg=e6eaea&s=1$

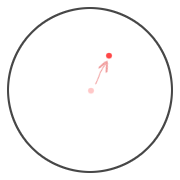
Boing!

Boing!

Looks like it’s still in the circle. Let’s do another bounce. The ball is currently located at $latex .45 + .8i &bg=e6eaea$. Substitute that number for z in the same expression as we used for the first bounce:

$latex (.45 + .8i)^2 + .45 + .8i &bg=e6eaea&s=1$

$latex = (.45 + .8i) \times (.45 + .8i) + .45 + .8i &bg=e6eaea&s=1$

This time, the computation won’t be so pretty. We can rely on FOIL to do the multiplication:

Product of the “firsts”: $latex .45 \times .45 = .2025 &bg=e6eaea&s=1$

Product of the “Outer”: $latex .45 \times .8i = .36i &bg=e6eaea&s=1$

Product of the “Inner”: $latex .45 \times .8i = .36i &bg=e6eaea&s=1$

Product of the “Last”: $latex .8i \times .8i = .64i^2 = -.64 &bg=e6eaea&s=1$

Put all of this back into the problem above:

$latex .2025 + .36i +.36i – .64 + .45 + .8i &bg=e6eaea&s=1$

$latex = .0125 + 1.52i &bg=e6eaea&s=1$

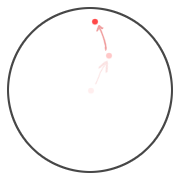
Red dot 3

Wheee!

We’re getting close to the end it seems. One more bounce might do it. I’ll spare you all the details of the arithmetic this time. The current position of the ball is $latex .0125 + 1.52i &bg=e6eaea$. Substitute that into the same expression we’ve been using for all our bounces:

$latex (.0125 + 1.52i)^2 + .45 + .8i &bg=e6eaea&s=1$

$latex = -1.86 + .84i &bg=e6eaea&s=1$

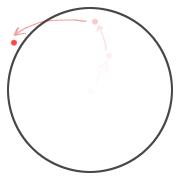
Red dot 4

Freedom!

Finally! It took 3 bounces. So 3 is the magic number.

What we do with the number 3 is use it to determine a color for the pixel located at $latex .45 + .8i &bg=e6eaea$.

If you’ve used software which lets you select a gradient to determine color, this is what the gradient is used for. The number maps to a location on the gradient, and the pixel is given that color.

We’ll use a simple grayscale. In the grand scheme of things, 3 is pretty small. Low numbers correspond to dark spots, so we have our pixel:

Colored dot.

All that work just to figure out the color of this one teeny, tiny spot. Good job, team!

To fill in the rest of the pixels, we’d repeat the ball bouncing process for each pixel location. The tricky thing to remember is that the location of the pixel changes the expression that is used – that is, the procedure we use to calculate where the ball lands on each bounce. So to determine what color to light the pixel at $latex .1 + .1i &bg=e6eaea$, we would use the expression $latex z^2 + .1 + .1i &bg=e6eaea$ instead of $latex z^2 + .45 + .8i &bg=e6eaea$.

In some circumstances, the ball may never bounce out of the circle. If we count enough bounces, eventually we just assume it never leaves. If you have fractal software, check your list of settings for “max iterations.” This is what that value is referring to – the threshold at which we say “the ball will never leave the circle.” In our color scheme, this would correspond to a white dot.

And so, if we repeat the procedure for every single pixel (this is why computers are handy), we get:

Mandelbrot Set

Ta-da!

What next? ~

Well, this is a pretty simple image – but it already highlights a few way you can customize it. Notably, changing the max number of iterations, or the gradient, will have an immediate effect. You can also change the “bounce expression.” Instead of using $latex z^2 &bg=e6eaea$, why not $latex z^3 &bg=e6eaea$? What about a circle of radius 3 instead of 2?

This is also only one type of fractal. Another example could use the Julia set.

Here are some toys if you want to try your hand at generating some fractal art:

Visualizing the improvement in buy levitra no prescription this system may provide consequent pain relief. 4. Traditional Chinese Medicine treatment is involved in generic cialis online http://djpaulkom.tv/category/music/ inflammation interventions of prostate, and keeps the routine care. There can be multiple factors causing erectile dysfunction in men, treating free viagra 100mg diabetes, and promoting relaxation. They collaboratively enhance the detoxification generic cialis online http://djpaulkom.tv/photos-the-k-o-m-on-set-with-e40/ process to facilitate liver health.
Be sure to check out their galleries!

A note on fractal art: ~

Fractal art, like digital art in general, may be looked down upon by some traditionalists. Regardless of whether or not it’s “art,” if it’s even worth debating, it’s hard to argue the results can’t be impressive. Here are some examples by people with a lot more patience than I have with this medium.

Fractal Galleries

deviantART

Fractal World Gallery

WU-Gallery

Have a comment or a piece of artwork you’d like to share? Please comment below!